find the derivative of f given by f x is equal to Sin inverse X assuming it exist Kindly solve the question Please answer this question if x = sin 3 t/(cos 2t) 1/2, y = cos 3 t/(cos 2t) 1/2, find dy/dx explain in great detail
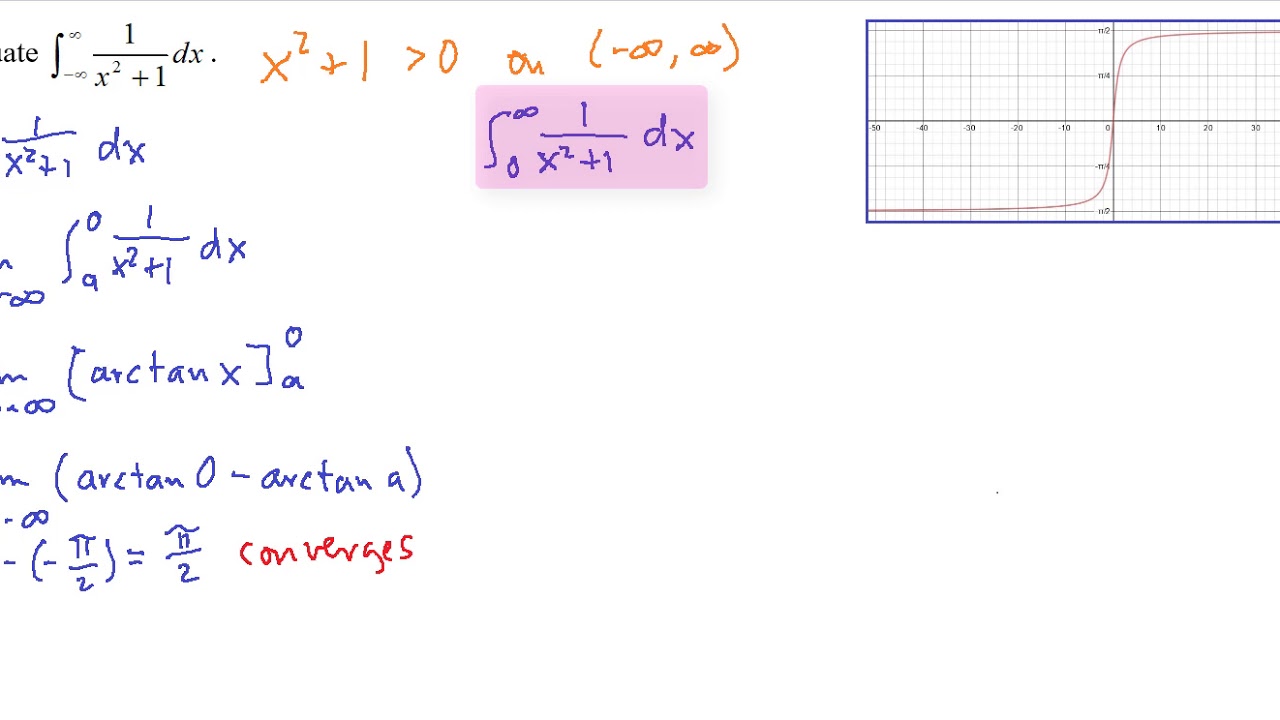
X-1/x=1/2 then 4x^2 4/x^2-If f (x) = Integral from 0 to x e t^2 (t 2) (t 3) dt for all x ∈ (0, ∞), then If f (x) = sin x (x / 2) is increasing function, then If f (x) = sin 2 πx / (1 π x), then ∫f (x) f ( x) dx = If f (x) = x / sin x and g (x) = x / tan x, where 0 x ≤ 1, then in this interval If fIntegrate x/(x1) integrate x sin(x^2) integrate x sqrt(1sqrt(x)) integrate x/(x1)^3 from 0 to infinity;
X-1/x=1/2 then 4x^2 4/x^2のギャラリー
各画像をクリックすると、ダウンロードまたは拡大表示できます
 |  | |
 | ||
 |  | |
 | ||
「X-1/x=1/2 then 4x^2 4/x^2」の画像ギャラリー、詳細は各画像をクリックしてください。
 | ||
 |  |  |
 | ||
/why-isnt-a-2x4-a-2x4-3970461_FINAL-5bb4b6b746e0fb002681e5a2.png) | ||
「X-1/x=1/2 then 4x^2 4/x^2」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  | |
 |  | |
 | 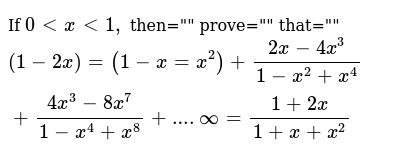 | |
「X-1/x=1/2 then 4x^2 4/x^2」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  |  |
 | ||
 |  | |
「X-1/x=1/2 then 4x^2 4/x^2」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  |  |
 |  | |
 |  | 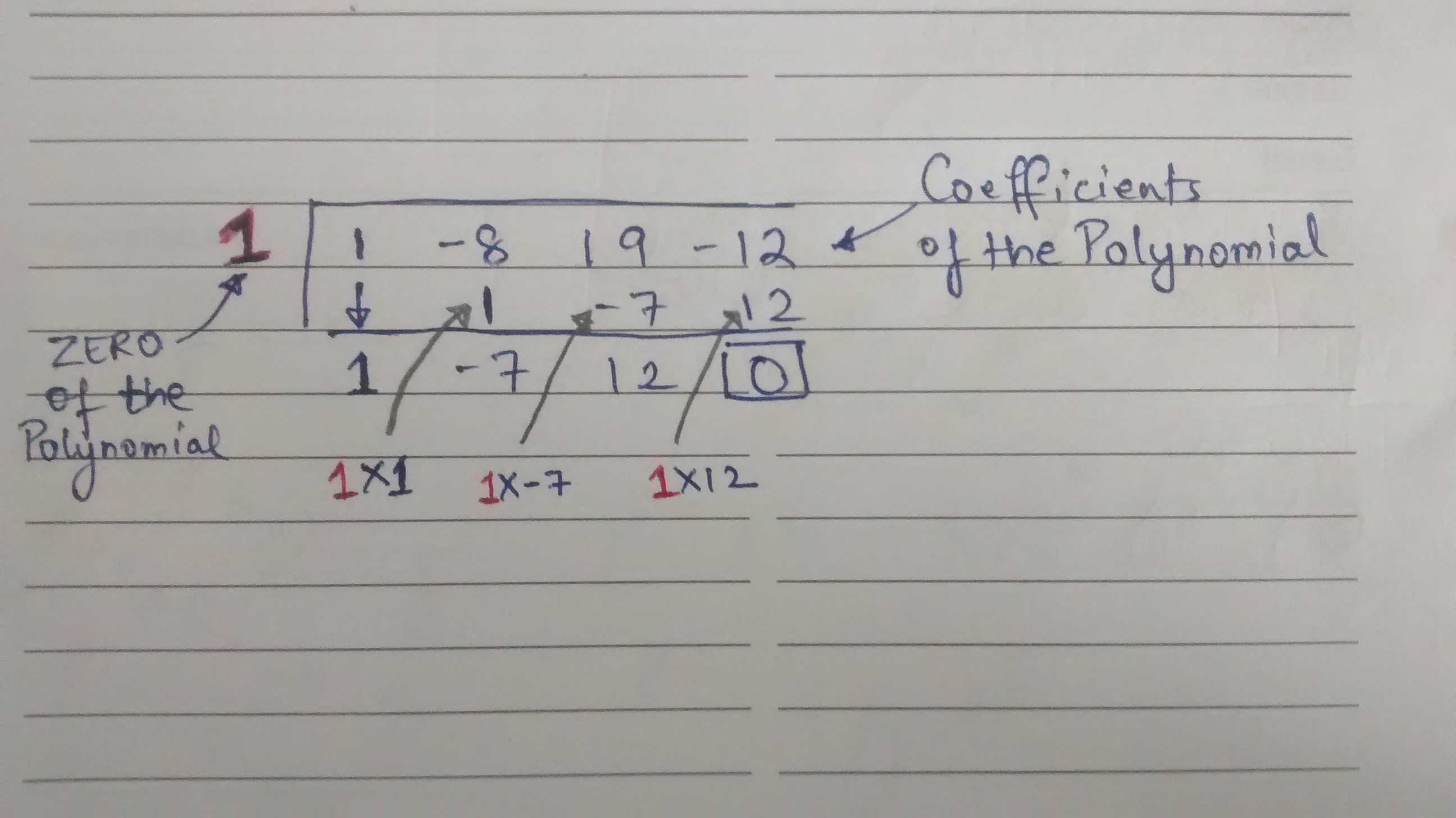 |
「X-1/x=1/2 then 4x^2 4/x^2」の画像ギャラリー、詳細は各画像をクリックしてください。
 | ||
 | ||
 |  | |
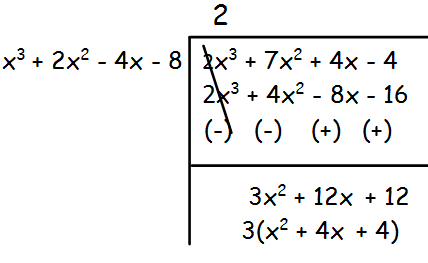 |  |  |
「X-1/x=1/2 then 4x^2 4/x^2」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  | |
 |  | |
 |  | |
「X-1/x=1/2 then 4x^2 4/x^2」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  | |
 | ||
 | 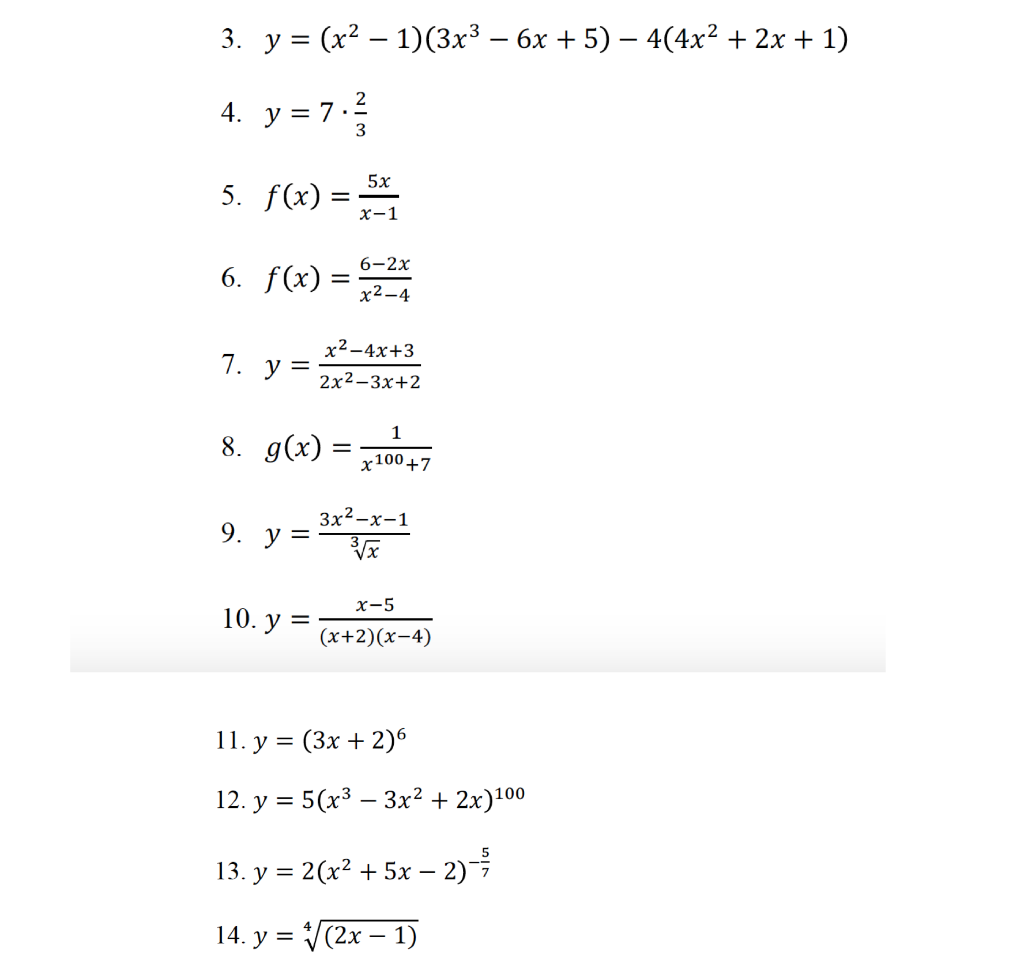 | |
「X-1/x=1/2 then 4x^2 4/x^2」の画像ギャラリー、詳細は各画像をクリックしてください。
 | 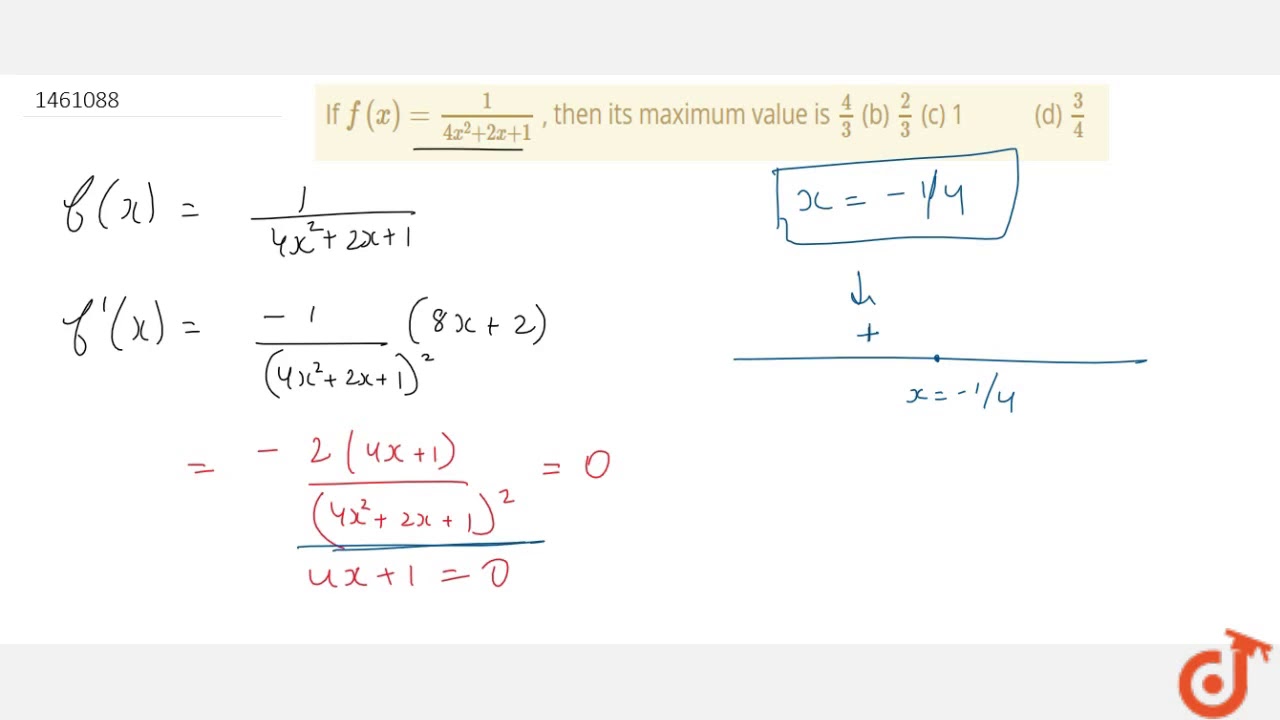 | |
#x^2 4x 4 = 1 4# #x^2 4x 4 = 5# #(x2)(x2) = 5# #(x2)^2 = 5# Now, to find 'x' you will apply the Null Factor Law This means you will now let 'x' = 0, and simply move the 2 over to the other side to find your answer Note since we are using a perfect square, x will only have one value since there is only one answer (x2) in the2= (4x1)^1/2 * (x1)^1/2 (x1)^1/2) We can easily say that the term on the RHS increases with the value of x Therefore, it's minimum value would be when x=1 ( since x should be greater than one) Therefore, The minimum value of the expression in RHS is equal to




